page1923 Rings Matthias Lorentzen...mattegrisenforlag.com
Look at the picture beneath, then
scroll down to the question and click the correct Answer button.
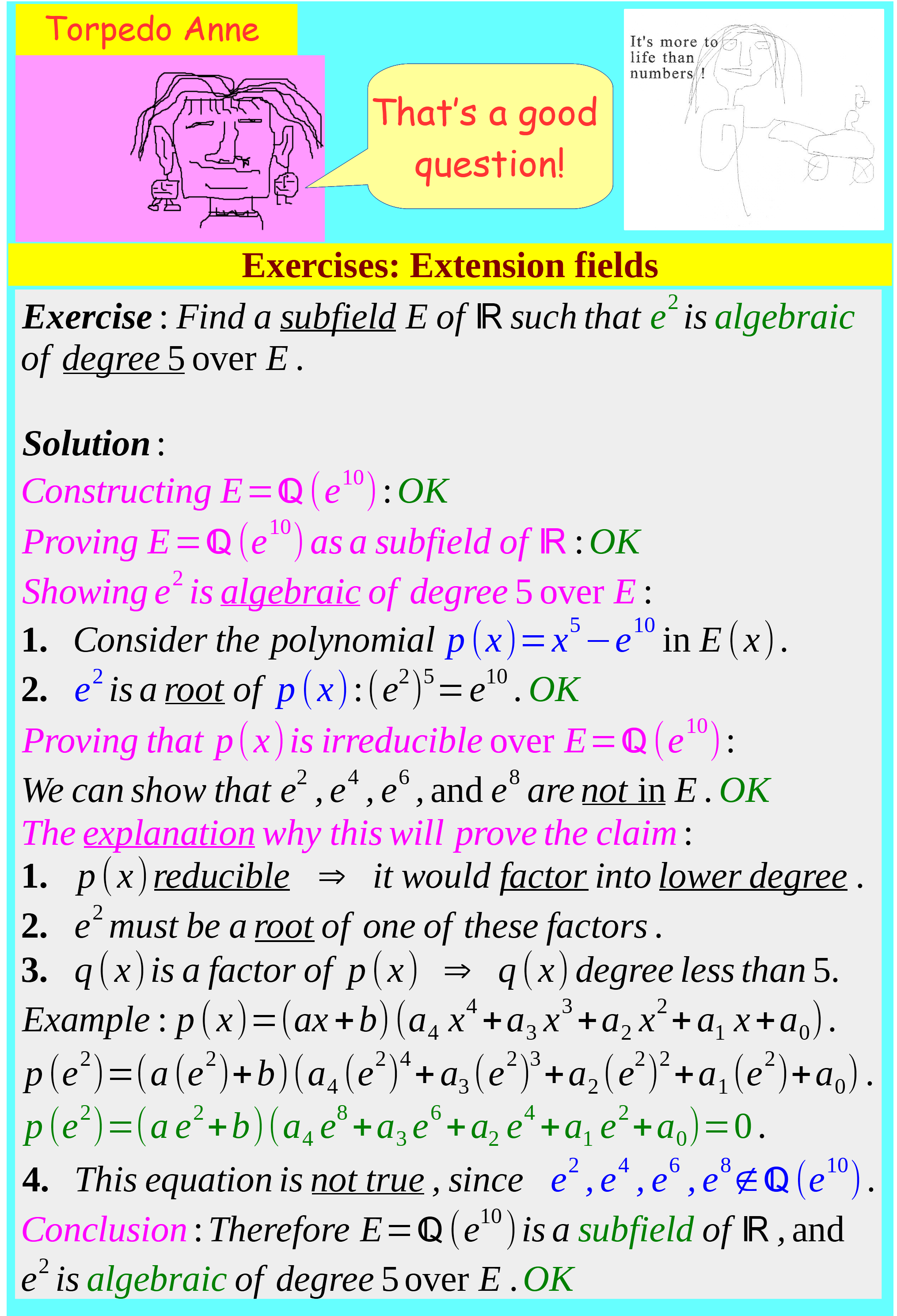
Question
Consider the field extension `QQ(e^10)` of `QQ`,
where e is the base of the natural logarithm.
Which of the following statements is true?
A) p(x) is reducible over `Q(e^10)` because
`e^2` is a root of p(x) and `Q(e^10)` contains
all rational functions of `e^10`.
B) p(x) is irreducible over `Q(e^10)` because
`e^2, e^4, e^6`, and `e^8` are not elements of `Q(e^10)`,
despite `e^2` being a root of p(x).
?